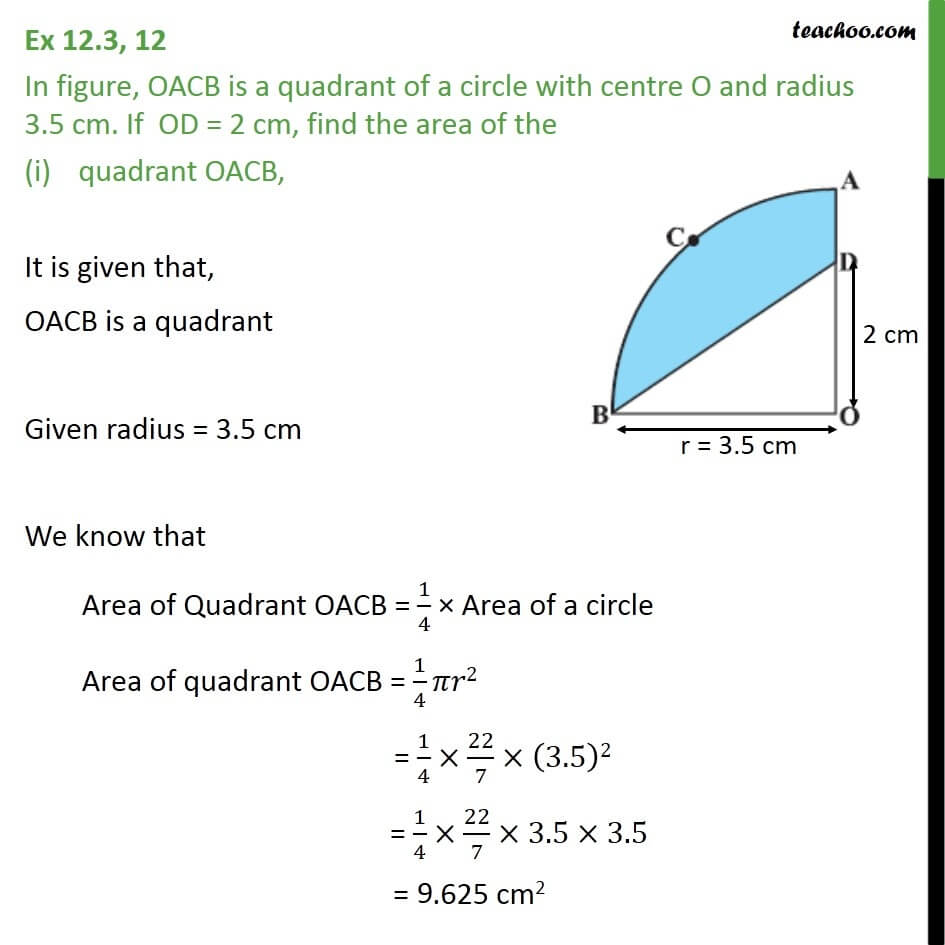
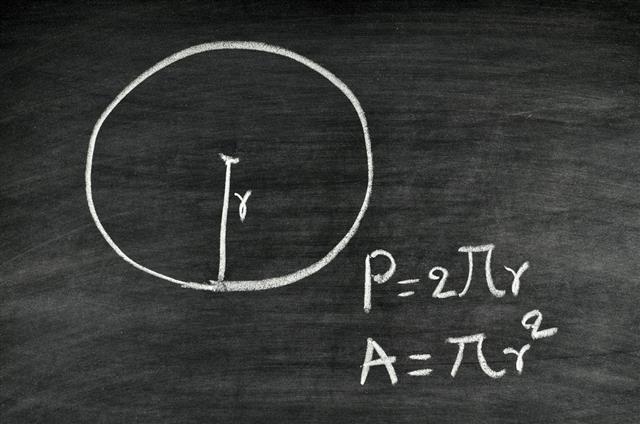As with triangles and rectangles, we can attempt to derive formulas for the area and "perimeter" of a circle. Calculating the circumference of a circle is not as easy as calculating the perimeter of a rectangle or triangle, however. A circle is a closed curve formed by a set of points on a plane that are the same distance from its center. The area of a circle is the region enclosed by the circle.
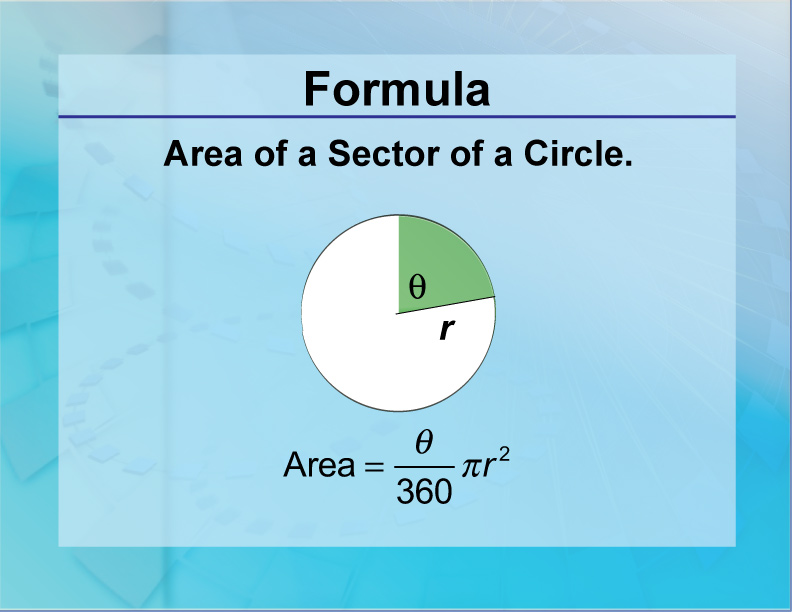
The area of a circle is equals to pi (π) multiplied by its radius squared. The formula for the area of a circle helps us calculate the area of circle sectors and segments as well. A circle sector's area in relation to the area of the whole circle is much like that between an arc and the circumference. A sector bound by a central angle of n degrees is equal to (n/360) times the area of the circle. The circle is divided into 16 equal sectors, and the sectors are arranged as shown in fig. The area of the circle will be equal to that of the parallelogram-shaped figure formed by the sectors cut out from the circle.
Since the sectors have equal area, each sector will have an equal arc length. The red coloured sectors will contribute to half of the circumference, and blue coloured sectors will contribute to the other half. If the number of sectors cut from the circle is increased, the parallelogram will eventually look like a rectangle with length equal to πr and breadth equal to r. The perimeter and area of triangles, quadrilaterals , circles, arcs, sectors and composite shapes can all be calculated using relevant formulae. A circle is known as a closed plane geometrical shape. Technically, it is the locus of a point that moves around a fixed point at a fixed distance that is away from that point.
Area Of A Circle Formula With Circumference A circle is basically a closed curve that has its outer line at an equal distance from the centre. This fixed distance from the central point is known as the radius of the circle. In our day to day life, we often see many examples like a pizza, wheel, etc. Let us learn about these terms in regards to a circle.
We use this formula to measure the space which is occupied by either a circular plot or a field. In this article today, we will discuss the area of circle definition, the area of a circle equation, its circumference and surface area in detail. In this lesson we learned about circles, which are shapes with all points the same distance from a center point.
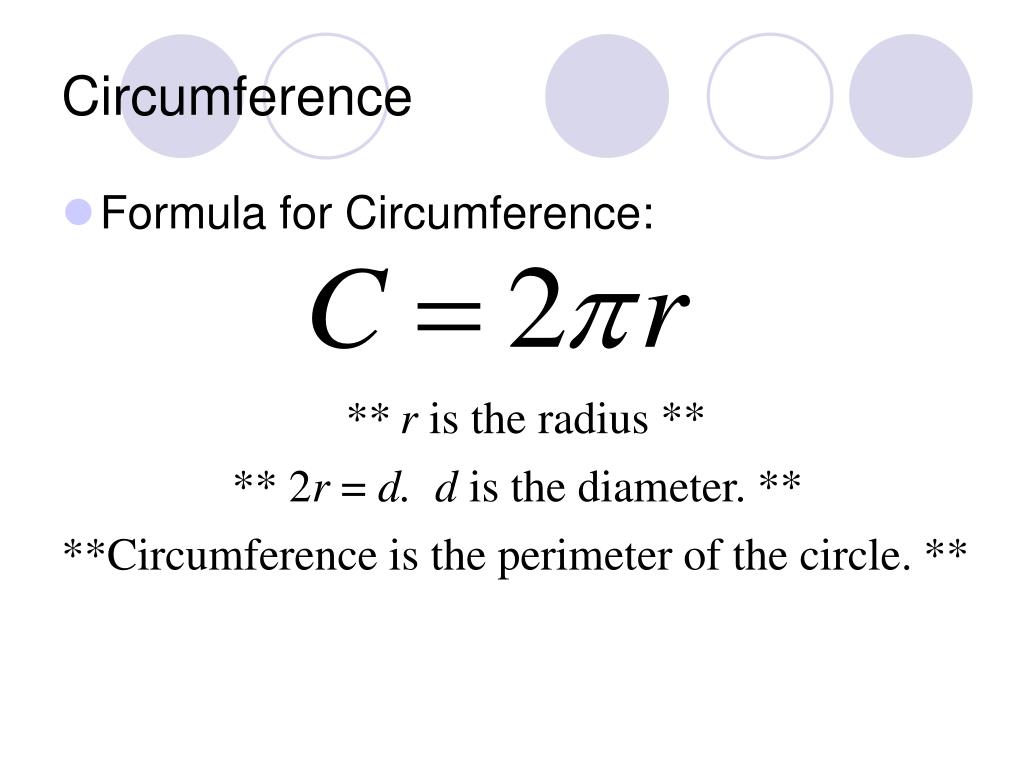
Circles are unique in that we call their perimeter a circumference. Various parts of circles, including radius, diameter, and chords, were introduced. Other parts such as the tangent, secant, and segments of a circle were also identified. There are two ways to find the circumference of a circle.
One circumference of a circle formula uses the diameter, while the other uses the radius. It is important to note that when calculating the circumference of a circle, neither the diameter nor the radius need to be squared. The area of a circle is the region or space measured inside the circle, or total number of square units inside the circle.
Calculating the area of a circle is not as straightforward as calculating the area of a square, for example, because of the object's shape. A circle may also have a semi-circle area which is half of a circle. It is derived by dividing the area of a circle formula by 2. When the length of the radius or diameter or even the circumference of the circle is already given, then we can use the surface formula to find out the surface area. This area is the region that occupies the shape in a two-dimensional plane.
Now we will learn about the area of the circle. So the area covered by one complete cycle of the radius of the circle on a two-dimensional plane is the area of that circle. Now how can we calculate the area for any circular object or space? In this case, we use the formula for the circle's area. In technical terms, a circle is a locus of a point moving around a fixed point at a fixed distance away from the point.
Basically, a circleis a closed curve with its outer line equidistant from the center. The fixed distance from the point is the radius of the circle. In real life, you will get many examples of the circle such as a wheel, pizzas, a circular ground, etc. Now let us learn, what are the terms used in the case of a circle. A circle is a closed shape formed by tracing a point that moves in a plane such that its distance from a given point is constant.
The word circle is derived from the Greek word kirkos, meaning hoop or ring. In this article, we cover the important terms related to circles, their properties, and various circle formulas. When we have the length of the diameter or the radius or the circumference of the circle, we can find the surface area of the circle by using the surface area formula. This surface area of the circle is represented in terms of square units.
The perimeter of a closed figure is known to be the length of its total boundary. When it comes to the circles, the perimeter is called by a different name. It is referred to as the 'circumference' of the given circle.
This circumference is known as the total length of the boundary of the given circle. If we open the circle and form a straight line, the length of the straight line that we get is the circumference. For defining the circumference of a circle, we need to know a term called 'pi'.
Consider the circle shown below having its centre at O and radius r. If you know the circumference, radius, or diameter of a circle, you can also find its area. Area represents the space enclosed within a circle. It's given in units of distance squared, such as cm2 or m2.
In order to find the area and circumference of a circle, one must know how to draw a circle. A circle is drawn as a closed arc around a center point. Circles have a constant distance between the center point of the circle and any point on the edge of the circle.
This distance is called the radius of the circle. The diameter of a circle is the distance of a line segment that runs from the edge of the circle to the opposite edge of the circle and must run through the center point. See the diagram for an illustration of these parts of a circle. For those having difficulty using formulas manually to find the area, circumference, radius and diameter of a circle, this circle calculator is just for you. The equations will be given below so you can see how the calculator obtains the values, but all you have to do is input the basic information. The distance from the centre to the outer line of the circle is called a radius.
It is the most important quantity of the circle based on which formulas for the area and circumference of the circle are derived. Twice the radius of a circle is called the diameter of the circle. The diameter cuts the circle into two equal parts, which is called a semi-circle. The perimeter of circle is nothing but the circumference, which is equal to twice of product of pi (π) and radius of circle, i.e., 2πr.
Fill the circle with radius r with concentric circles. After cutting the circle along the indicated line in fig. 4 and spreading the lines, the result will be a triangle.
The base of the triangle will be equal to the circumference of the circle, and its height will be equal to the radius of the circle. A perimeter of closed figures is defined as the length of its boundary. When it comes to circles, the perimeter is given using a different name.
It is called the "Circumference" of the circle. This circumference is the length of the boundary of the circle. If we open the circle to form a straight line, then the length of the straight line is the circumference. To define the circumference of the circle, knowledge of a term known as 'pi' is required. Hence, the concept of area as well as the perimeter is introduced in Maths, to figure out such scenarios. But, one common question that arises among most people is "does a circle have volume?
Since a circle is a two-dimensional shape, it does not have volume. In this article, let us discuss in detail the area of a circle, surface area and its circumference with examples. The circumference of the circular water pit is 18 feet. How can Marcus use this information to determine how long the beam needs to be to go across the center of the pit?
Marcus needs to figure out the diameter of the pit. Π is simply a ratio between the diameter, d of a circle and it's circumference. In other words, a circle that has a diameter of 1 or a radius of 1/2 will have a circumference of π. Π is conveniently also the ratio of r squared to the area of the circle, meaning that a circle with radius 1 will have an area of π.
Let's take a look at some of your questions. In this method, we divide the circle into 16 equal sectors. The sectors are arranged in such a way that they form a rectangle. All sectors are similar in area, so hence all sectors' arc length would be equal.
The circle's area would be the same as the area of the parallelogram shape or rectangle. This lesson also helped to determine the circumference of a circle and how to calculate its area using radius or diameter. The circumference is the distance around the edge of the circle. The area of a circle is the amount of two-dimensional space that the circle takes up. Formulas can be easily used to find both the area and circumference of a circle. The area of the circle is the measure of the space or region enclosed inside the circle.
In simple words, the area of a circle is the total number of square units inside that circle. To recall, the area is the region that occupied the shape in a two-dimensional plane. In this article, you will learn the area of a circle and the formulas for calculating the area of a circle.
Since area is a measure of two dimensions, you always report area in square units like square inches or square feet . This is especially important when calculating the area of a circle for an assignment since an answer without correctly reported units is likely incorrect or incomplete. We have discussed till now the different parameters of the circle such as area, perimeter or circumference, radius and diameter. Let us solve some problems based on these formulas to understand the concept of area and perimeter in a better way. As we know, the area of circle is equal to pi times square of its radius, i.e. π x r2. To find the area of circle we have to know the radius or diameter of the circle.
Fizzywoz - If you look at examples #1-#4 at the end of the article you can see how numbers are plugged into the equation. You will usually just plug in a known radius into the equation to figure out the circumference or you may plug in a known circumference to determine the radius. Let me know if you have any other questions.
Is made up of a large number of concentric circular pieces of very thin string. Finding the radius is not always easy, especially if you don't have the circle's center. You can calculate the area using the diameter instead. The same formula applies as above, but you need first to calculate the radius of the circle. Simply divide the diameter by 2 to get the radius. The circle circumference is the perimeter of an elliptical or circular shape.
In other words, this is the arc length or boundary length of the circle; if we straightened it out or open up in a line segment. In this image, you can see 16 sectors, including 8 green and 8 blue ones. The green highlighted sectors represent the circle's half circumference while the other half of circumference is represented by blur highlighted ones. By increasing the number of the sectors cut from the circle, the parallelogram will change into a rectangle. The length of the rectangle would b equal to πr with a width equal to r. Only a mathematician can genuinely understand the practical importance of formulas for calculating area, radius, diameter, or circle circumference.
While most people think that formulas have no practical use, they are critical factors in many everyday life routines. The area of a circle is any space that the circle occupies on a flat surface. When we talk about the surface area of the circle, we are focusing on two-dimensional objects. When finding the circle area, there are three other measures that we take into consideration, including the circumference, diameter, and radius. All three calculations also help us fining the circle area.